Magic

The Sagrada Familia is a truly amazing site to behold. Designed by Gaudi, and towering over a corner of Barcelona, it has been under construction for over one hundred years. Progress is slow to say the least, but maybe it will be finished in our lifetimes.

We went there on our summer holiday in 2006 as Barcelona was a stop on our first Med cruise, aboard Legend of the Seas. We'd signed up for the Tapas tour, and before we got stuck into the food and drink, our first stop was for a quick look at the famous cathedral.

Unfortunately, being on a guided tour with an itinerary to keep to, we didn't have time to go in, so had to make do with taking a few photos from the outside. Whilst I do enjoy a bit of tapas, I could quite happily have stayed there all day. Must go back and do that sometime soon.

One of the sights that impressed me most was that of the Passion Facade, a comparatively recent addition to the building. In a series of sculptures forming an 'S' shape, from bottom to top it tells the story of the Passion of Christ.

Just to the left of the door is a depiction of Jesus being kissed by Judas, and next to the figures, on the wall, is a square which contains a 4x4 grid of numbers.

This is a magic square. Add up any row, column or diagonal on the square and the total comes to 33, believed to be Jesus's age when he was crucified.

The magic square in Barcelona is actually a slightly amended version of another famous square. You will notice that in the square above, two numbers appear twice, 10 and 14. If you add 1 to the 14 on the right, and add 1 to the 10 on the left and then rotate the whole thing by 180 degrees, you end up with this:

This is also a magic square. Also, it is called a 'normal' magic square because it features each of the numbers from 1 to 16.

(I think that the left hand column is a bit hard to read, so for avoidance of doubt, from top to bottom it is 16, 5, 9, 4. To me, the 5 looks to be upside down, and also if you look closely I can't help but wonder whether it is done that way to cover up a mistake - looks like there might once have been a 6 there. Also, the 9 looks more like a question mark.)

This square is known as Durer's magic square, and this is because the image above is an extract from an engraving made almost half a millennium ago by Albrecht Durer. In fact the date of the engraving is 1514, which can be seen in the middle of the bottom row of the square, with 4 and 1 on either side (the artist's initials being the first and fourth letters of the alphabet).

The square in the engraving isn't just magic, it is very magic!

As in the Barcelona square, adding up all of the rows, columns or diagonals (i.e. 10 different possibilities) gives the same answer, in this case 34.

(Note that all normal 4x4 magic squares share this property. If c is the magic constant, then we know that the total of all of the numbers in the square, that is the sum of each row, must be 4c. But also, the sum of the four rows must also be the sum of the numbers from 1 - 16 which is (1/2 x 16 x 17) 136. So if 4c = 136 then c = 34.)

But, it isn't just rows columns and diagonals that add to 34. It is also the case that if you add the four digits making a 2x2 square in the top left hand corner, that adds up to 34. The same applies to the top left, bottom left, and bottom right 2x2 squares, as well as the 2x2 square in the middle of the main square. The answer can also be achieved by adding the squares in the corners of the Durer square (that's another 6 possibilities).

Now, take a look at the picture below:

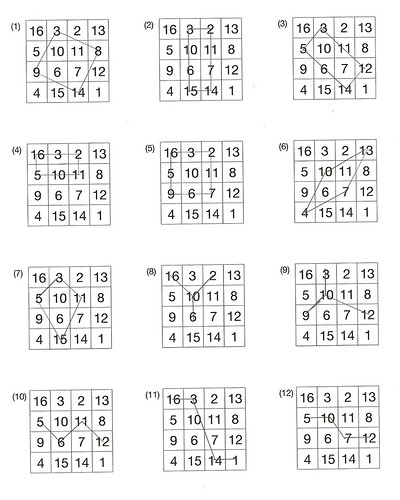
Each of the twelve examples above gives more ways to find 34. Taking each numbered square above in turn:

1. The four numbers in the corners of the slanted square drawn here add to 34. Similarly, there is another slanted square (2, 5, 15, 8) that works (that's 2 more possibilities).
2. The four numbers in the corner of the rectangle add to 34. Rotate the rectangle 90 degrees and it works again (another 2 possibilities).
3. Again add the corners of the rectangle, this time a diagonal one, to get 34. Also rotate by 90 degrees for another (2 more).
4. Yet again, corners of the rectangle add to 34, and there are three more like this (by moving the rectangle either one space to the right, or down two, or both). Also, if you stand the rectangle up so that its corners are on 16, 3, 9 and 6, then we still get 34. Similarly there are four of these stood up rectangles made by moving the first one either down one, across two, or both (that's another 8).
5. Sum of the corners of the square is 34. There are four such squares made by starting in any of the four corners of the Durer square (4 more).
6. The sum of the points of the diamond is 34. Another one can be made by going top left to bottom right (another 2).
7. Kites. There are four kites whose corners add to 34 - the one pictured, plus one moved one space to the right, plus the two made by rotating those about a horizontal axis (that's another 4).
8. The sum of the the ends of the tripod plus the square in which all the lines meet is 34. Four different tripods can be made using the exact same operations as for number 7 (4 more).
9. Uneven tripods work as well. The one shown above, plus another three made by rotating in horizontal or vertical axes or both (another 4).
10. The zigzag adds to 34. So do the other three made by rotating and/or reflecting. Also, if you move the top two numbers up one and the bottom two you get 16, 15, 2, 1 which is a more pronounced zigzag that adds to 34. Again there are four of these made by rotating and reflecting (8 more).
11. The two pairs shown in the diagram add to 34 and once again there are three more similar examples made by rotation and reflection (so we get another 4).
12. Finally, just like for 11 above there are four ways to make this shape that adds to 34 (4 more).

If we add up all of the highlighted numbers in the list above and the paragraphs above that, we've found 64 ways of making a total of 34 whilst also making a pattern on the square.

How many ways are there to make a total of 34 from the numbers in the square, irrespective of whether they make a pattern or not? I think that the answer to that is 86.

(Dad - need your help here as I haven't proved that, I've just written them all down! There are 1,820 ways of picking 4 from the numbers 1 to 16 (16! / 12! x 4!). The smallest possible answer is 10 and the largest is 58, so the most common answer will be 34. Knowing this much, along with, I presume, the possible sums being normally distributed, is there an easy way to prove this?)

So, we've shown that the majority of possible ways of finding 4 choices from the square such that they add to 34 are arranged to make a pattern within Durer's square.

Now that's magic.